OSSD中的排列小知识之PERMUTATION是什么东东呢?
2020-05-27 环球教育
今天呢,我们要来聊一聊关于OSSD中的排列小知识之PERMUTATION
首先让我们看一个简单的英文小事例
In arranging seven students to sit in the front row for a class picture,the photographer first puts the three girls to the right and the four boys to the left.He is not pleased with this setup.Then he tries mixing boys and girls,starting with Brian at the far left.He feels this is still not quite right.Then he rearranges the group so that the tallest,Donna,is at the left with the shortest,Shawn,at the right.Still he is not satisfied!How many possible arrangements of the seven students are there?

在生活当中我们经常会碰到关于排列组合的事情,比如有个摄影师想要安排七个学生坐在前排拍摄照片,摄影师首先将三个女孩放在右边,四个男孩放在左边,他对此设置不满意,然后他尝试从最左边的brain开始混合男孩和女孩,他觉得这还是不太正确,然后他重新排列组合。最高的Donna站在左边最矮的shawn右边,但是他还是不满意,那么我们可以想一想,这七个学生其实有多少种可能的安排组合呢?
In order to apply the Fundamental Counting Principle,we reason as follows:
The position for the students can be illustrated by using a series of seven boxes.
为了应用基本计数原则,我们可以这样:
使用七个盒子系列可以说明学生的位置。
In the position at the far left,there are seven possibilities because any student could be put there.In the next position there are six possibilities because the student in the first position cannot also be in the second position.This reasoning continues so that there are:7×6×5×4×3×2×1=5040ways of arranging these students.
Let's have a look at more examples below:
在最左边的位置,有七种可能性,因为任何学生都可以被放在那里。在下一个位置有六种可能性,因为处于第一位置的学生也不能在第二位置。这种推理继续下去,以便有:7×6×5×4×3×2×1×5040的方式安排这些学生。
When we count the number of different arrangements where order does matter,we call this a PERMUTATION.
当我们计算顺序很重要的不同安排的数量时,我们称之为"排列”。
其实不论在那里学习数学,用哪种语言接触数学,我们学习到的东西都是相通的,而且总可以有一种我们汉字相对应的解释,大家完全不用担心哦~
那么留一个小练习,检查你对排列是否有一些了解呢?
你能给词语“MATTER”进行字母的排列组合吗?
上一期关于杨辉三角形,我们留了一道小练习,现在公布一下答案哟~
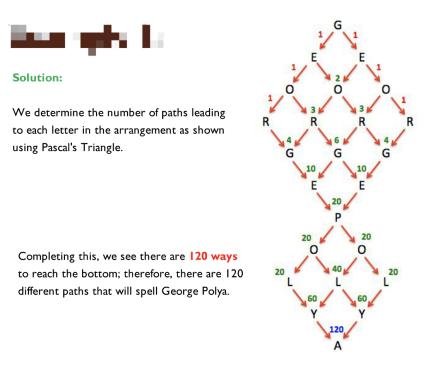
下期见呦~
更多OSSD资讯可持续关注http://beijing.gedu.org


1 秋季雅思培训班全面解析:多元课程体系+精准提分策略,助你高效冲刺目标分数
2 秋季雅思培训班全面开课:分层教学适配多元需求,抢占备考黄金期
3 秋季雅思 4 分培训班报名开启:夯实基础,轻松拿下入门目标分
4 秋季雅思6.5分冲刺攻略:课程选择与高分策略全解析
5 雅思秋季班报名启动:封闭集训、OMO混合课程成新趋势
6 出国雅思培训班:为留学之路筑牢语言基石
7 雅思备考进入「精准提分」时代:从痛点到破局,2025年考生需要怎样的培训?
8 雅思课程培训:体系化学习,高效突破分数壁垒
9 2025雅思培训班避坑指南:如何选择高性价比机构?
10 2025雅思口语培训新生态:动态题库下的高分突围指南
北京市海淀区环球雅思培训学校 版权所有 课程咨询热线:400-616-8800
Copyright 1997 – 2025 gedu.org. All Rights Reserved 京ICP备10036718号
全部课程、服务及教材面向18岁以上人群
市场合作申请