OSSD反函数的概念
2020-05-27 环球教育
大家好,今天我们来聊一下OSSD反函数的概念。
Inverse functions are a special class of functions that undo each other. The input and output values for two inverse functions, f(x) = 2x + 1 and g(x) = x-12 , are shown below:
反函数是一类相互撤销的特殊函数。两个反函数的输入和输出值如下所示:
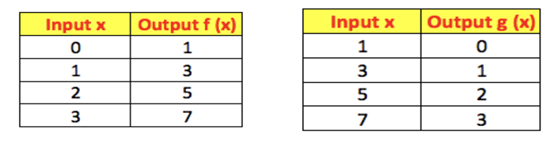
Notice that the output of the first function, f(x), becomes the input for the second function, g(x). The function g(x) undoes what f(x) does. The ordered pairs of g(x) can be found by switching the coordinates in each ordered pair of f(x).
Recall that a relation is a set of ordered pairs. The inverse of a relation can be found by interchanging the domain and the range of the relation:
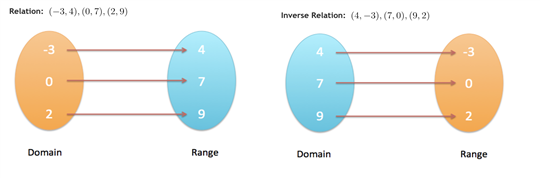
请注意,第一个函数f(x)的输出成为第二个函数g(x)的输入。
回想一下,关系是一组有序对。反函数关系可通过互换关系的域和范围来找到。
You will also recall that a function is a special relation. For each element in the domain of a function, there is exactly one element in the range. If the inverse of a function f(x), is also a function, it is called the inverse function of f(x). The inverse function is represented by f-1(x).
The notation of f-1 is read as 'the inverse of f' or simply 'f inverse'. Please note that -1 is not an exponent(指数), therefore, f-1≠ 1f
The table shows ordered pairs belonging to a function f(x). Determine f-1(x), graph f(x) and its inverse and state the domain and range of f(x) and its inverse.
下表显示了属于函数f(x)的有序对。接下来我们确定 f-1(x),图及其逆,并说明其逆的定义域和值域。
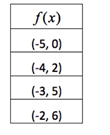
In this example, switch the x and y coordinates, and then plot the points.
在此示例中,切换x和y坐标,然后绘制点

Notice that switching the x and y coordinates reflects the graph of f(x) in the line y = x.
请注意,切换和坐标会转换直线中的x与y关系
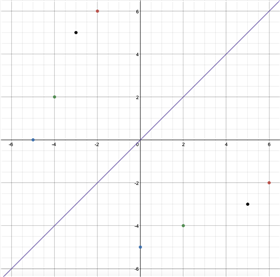
Notice below that the domain of f(x) is the range of f-1(x) , and the range of f(x) is the domain of f-1(x) :
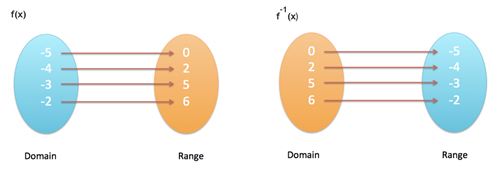
请注意,上图中f(x)的定义域是f-1(x) 的值域,f(x)的值域是f-1(x) 的定义域。这就是求f(x)反函数的方法。
更多OSSD知识可以持续关注http://beijing.gedu.org


1 秋季雅思培训班全面解析:多元课程体系+精准提分策略,助你高效冲刺目标分数
2 秋季雅思培训班全面开课:分层教学适配多元需求,抢占备考黄金期
3 秋季雅思 4 分培训班报名开启:夯实基础,轻松拿下入门目标分
4 秋季雅思6.5分冲刺攻略:课程选择与高分策略全解析
5 雅思秋季班报名启动:封闭集训、OMO混合课程成新趋势
6 出国雅思培训班:为留学之路筑牢语言基石
7 雅思备考进入「精准提分」时代:从痛点到破局,2025年考生需要怎样的培训?
8 雅思课程培训:体系化学习,高效突破分数壁垒
9 2025雅思培训班避坑指南:如何选择高性价比机构?
10 2025雅思口语培训新生态:动态题库下的高分突围指南
北京市海淀区环球雅思培训学校 版权所有 课程咨询热线:400-616-8800
Copyright 1997 – 2025 gedu.org. All Rights Reserved 京ICP备10036718号
全部课程、服务及教材面向18岁以上人群
市场合作申请